Pi may be a famous irrational number, but there is nothing irrational about celebrating it with your students! Even though there are infinitely many irrational numbers, very few of them have infiltrated popular culture—and none in the way that pi has, with memorization competitions, a perfume, and even an eponymous movie. It probably doesn’t hurt either that “pi” sounds like “pie,” making way for an especially tasty mathematical celebration.
What Is National Pi Day?
Pi Day is a yearly celebration of the mathematical constant \(\pi\). Pi Day dates back over 30 years to 1988, where Pi Day was first celebrated at a large scale in San Francisco’s Exploratorium by physicist Larry Shaw. The holiday has only picked up steam since then, with the recognition of Pi Day gaining support by both the US House of Representatives in 2017 and—this makes it international Pi Day!—the United Nations in 2019. Today, math teachers around the country celebrate Pi Day in the classroom.
When Is Pi Day in 2025?
PI Day is observed every year on March 14th. March 14th is always Pi Day since the date 3/14 resembles the first few digits of pi: 3.14. March 14th also happens to be Albert Einstein's birthday.
What Day Is Pi Day in 2025?
In 2025, March 14th is a Friday. If you’re in class at 1:59 PM, you can even celebrate Pi Minute, which is the first six digits of pi: 3.14159.
Why Do We Celebrate Pi Day?
For many teachers, Pi Day is a big chance to bring celebration into the math classroom. How often does math get a holiday?
Many teachers celebrate the 100th day of school, and we certainly won't stop you from celebrating events like Math Storytelling Day (September 25) or—why not—Katherine Johnson’s birthday (August 26). I would add Albert Einstein’s birthday to the list, but it already falls on Pi Day!
However, the illustrious history of pi spans millennia and has more than earned pi’s status as a holiday. Its relationship to the circle makes it approachable to students just starting school, and its qualities as an irrational number can make it captivating to math students of all ages.
What Do You Do on Pi Day? How to Celebrate Pi Day in the Classroom
Here are some fun ways to celebrate Pi Day in the classroom, particularly for younger children.
- Celebrate with Pie: This is the way that Pi Day has been celebrated since its inception! Pi and pie both share a relationship to the circle. Consider making \(\pi\)-shaped pies or decorating a pie with digits of pi for the numerical festivities.
- Calculate Pi as a Class: Have each student find a circular object, then measure the circumference (string often helps) and diameter. What do they get when they divide the two measurements? If you average everyone’s calculations, how close do you get to pi?
- Hunt for Pi: We have a pi scavenger hunt designed for middle schoolers, but here we offer one more scavenge. Prepare a few texts that are not part of your current math materials that contain the number \(\pi\) somewhere. It is not important that students understand the text. Have them find the \(\pi\). Facilitate a conversation about the number. How is it notated differently? Is it part of a fraction? What do your students notice or wonder?
However, this is not the only Shaped article to discuss what to do on Pi Day. We already have a few to help you and your students out, which span different grades and interests:
What Is Pi?
Let’s take a step back. What is this number that has been exalted with its own holiday? To start, pi is the English spelling of the Greek letter \(\pi\) and is usually pronounced in English identically to “pie.” In math class, it is just a number. In a certain light, it’s no more special than 2 or 78.163. But let’s pause on those numbers. The number 2 appears all the time. You’ve probably had to count to 2 within the last hour! What about 78.163 though? Can you even say with confidence that you’ve ever experienced that number before in your life? It sits just as innocently on a number line as 2, yet it doesn’t have the same utility.
So how did this business of \(\pi\) come about? It’s even harder to pinpoint on a number line than 78.163, yet the number \(\pi\) actually does show up from time to time—in school, on TV, in the news...anywhere someone might be talking about math or science. This is because the number \(\pi\) has an important relationship to a circle.
If you ask students what they can measure about a circle, two common responses are the circumference and the diameter, though they may not use those words. We can teach this enigmatic number even to very young children because of this connection.
When you “unroll” a circle, you get a length a little more than 3 times the diameter; this is \(\pi\). In other words, \(\pi\) equals the ratio between the circumference and diameter of a circle. Mathematicians since the dawn of history have been trying to calculate just what is that number?

Nowadays it’s a well-known fact that the number is \(\pi\) about 3.14. But oh, there is so much wrapped into that word “about.” First off, it’s not exactly any decimal you can write. This is probably the fact for which it’s most famous. It’s not exactly equal to 3.14. Or 3.1415. Or 3.14159265358979. It’s what’s known as an irrational number because the decimal expansion never repeats or ends. However, \(\pi\) is not the only irrational number. In fact, there are in a way even more irrational numbers than rational numbers! So what makes \(\pi\) so special?
Approximating Pi with Polygons
In our post about the history of pi, we step through the global origins of the number. People who lived millennia ago understood that no matter how big the circle is, the circumference and diameter always form the exact same ratio.
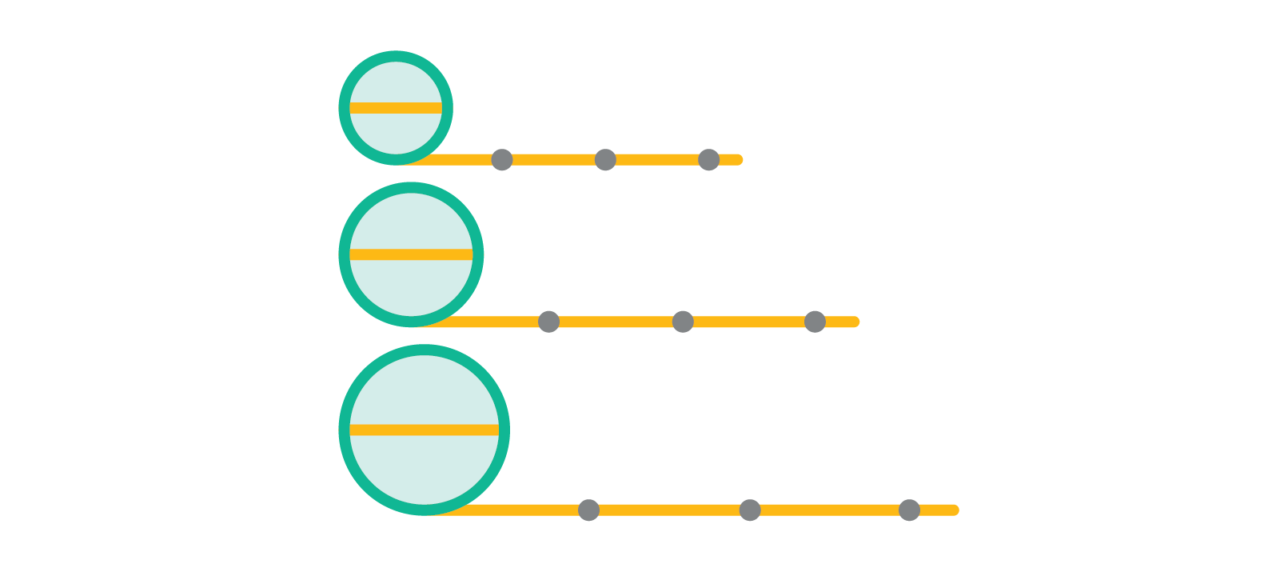
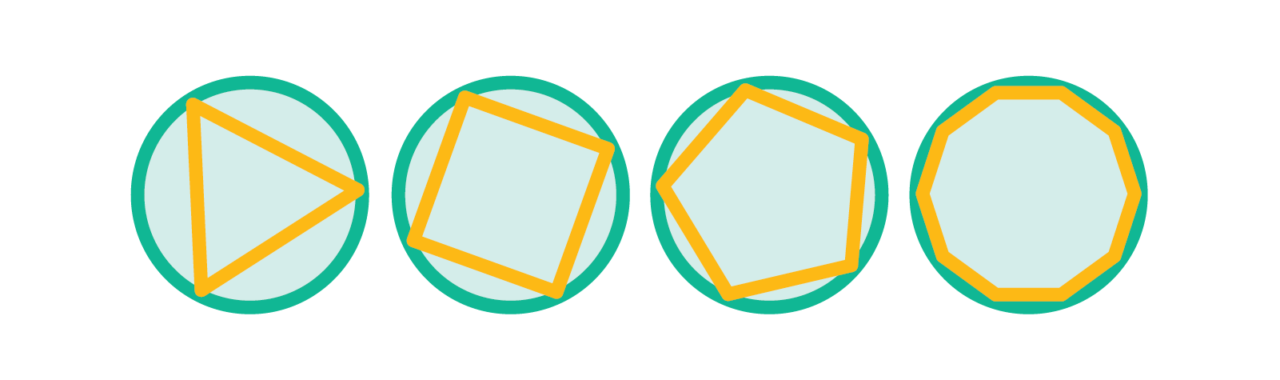
The diameter is straightforward to measure. Hold up a ruler. Even before there were rulers, one could measure it using sticks or hands. But measuring the circumference is trickier. You can wrap a string around the circle, then straighten out and measure the string...but that’s not exact. The quest to compute just what \(\pi\) is started out by approximating circles using polygons whose perimeter we could measure. The more sides the polygon has, the closer it is to a circle:
Calculating Pi with Polynomials
By ”polynomials,“ I mean the transition from geometry to algebra. Instead of hypnotizing polygons within circles, we get austere polynomials like this:
\[x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}+\frac{x^9}{9}-\cdots\]
A breakthrough was figuring out ways to calculate \(\pi\) using formulas. The developments of algebra and calculus gave way to exact formulas that can produce arbitrarily precise decimal expansions of \(\pi\), such as this one, known as the Leibniz, Gregory, and Nilakantha formula for pi:
\[1-\frac{1}{3}+\frac{1}{5}-\frac{1}{7}+\frac{1}{9}-\cdots=\frac{\pi}{4}\]
Today \(\pi\) is used all throughout math and science. There are some formulas involving circles that are used constantly, such as the area, \(A\), or circumference, \(C\), of a circle. Here, \(r\) is the radius of the circle:
\[A=\pi r^2\]
\[C=2\pi r\]
There are also formulas for 3-D shapes like the volume, \(V\), or surface area, \(S\), of a sphere. Here, \(r\) is the radius of the sphere:
\[V=\frac{4}{3}\pi r^3\]
\[S=4\pi r^2\]
However, virtually every pocket of math has \(\pi\) buried in it somewhere! There is a famous equation called Euler’s identity that combines \(\pi\) with 0, 1, the irrational constant e, and the imaginary constant i into one formula:
\[e^{i \pi}+1=0\]
The number \(\pi\) is found all throughout science, too. Because \(\pi\) is the ratio between the circumference and diameter of a circle, it appears when translating between linear and circular distance, or in other words, how far a wheel travels. Engineers use \(\pi\) when building an odometer. Astronauts care about \(\pi\) when planning the paths of spacecrafts orbiting moons and planets. And geologists care about \(\pi\) when understanding the composition and shape of Earth.
Sometimes, the circles are a little more hidden. Consider the following formula, known as Coulomb’s law, for calculating the electrostatic force, \(F\), between two particles. Here, \(q_1\) and \(q_2\) are charged particles, \(\varepsilon_0\) is known as the electric constant, and \(r\) is the distance between the particles:
\[F=\frac{|q_1| |q_2|}{4\pi \varepsilon_0 r^2}\]
Okay, this article is definitely not about electromagnetism. My point is this: the \(\pi\) in the denominator is part of a bigger story. Physically speaking, the electricity from a point in space radiates outward in all directions from that point. A sphere! Even though there are no obvious circles in measuring electricity, spheres are “hidden” in the calculation and cause a \(\pi\) to appear.

Ready to commemorate Pi Day with your students? Think of the holiday as a chance to think like mathematicians and look critically at how numbers can relate to shapes. There are still so many unsolved problems involving pi. Perhaps your students will one day solve a few!
***
Find rich math activities to help you figure out how to celebrate Pi Day throughout our PreK–12 math solutions.
Download our FREE calendar of activities!














